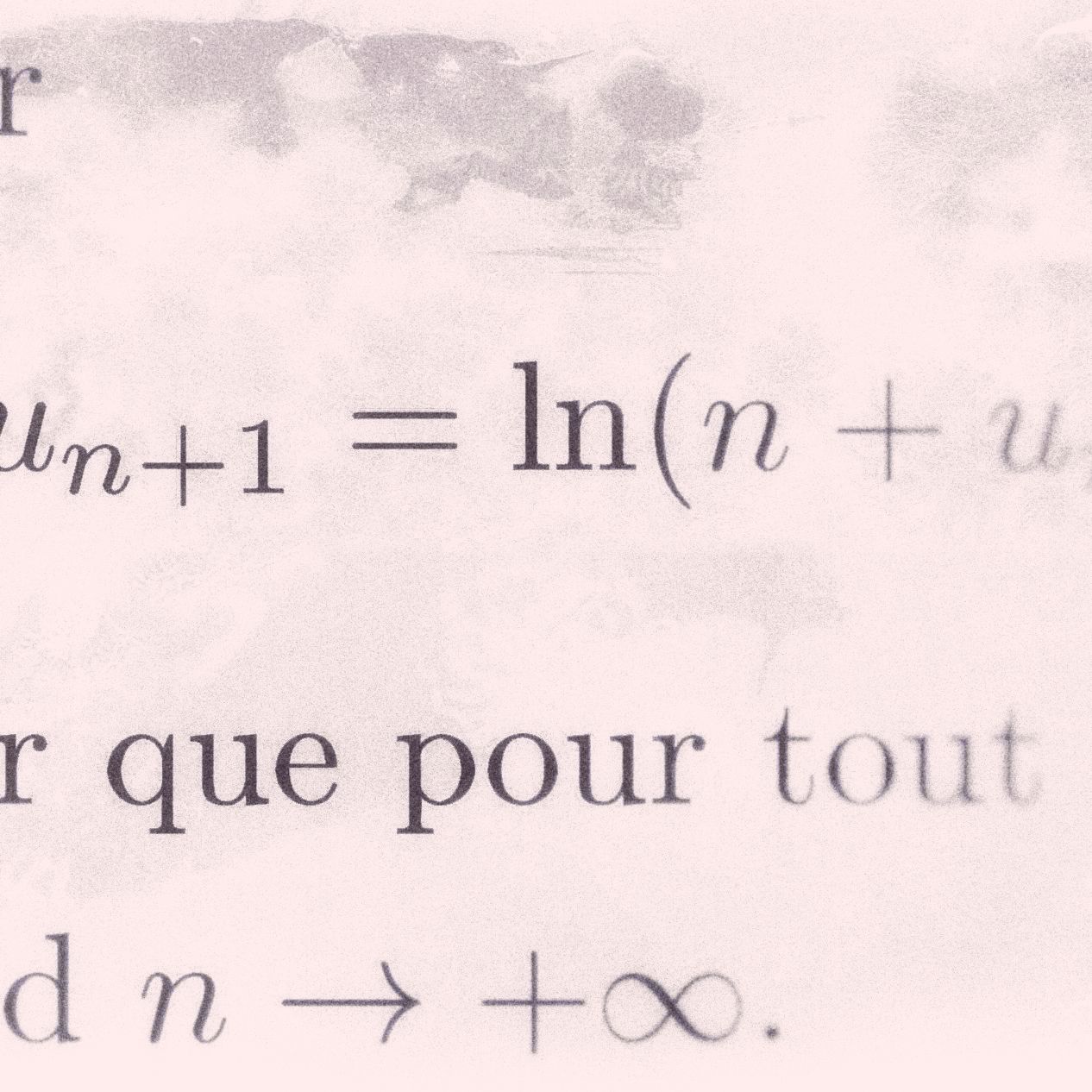\(\newcommand{\eps}{\varepsilon}
\newcommand{\llbracket}{[\![}
\newcommand{\rrbracket}{]\!]}
\newcommand{\D}{\mathrm{D}}
\newcommand{\C}{\mathbb{C}}
\newcommand{\Q}{\mathbb{Q}}
\newcommand{\R}{\mathbb{R}}
\newcommand{\U}{\mathbb{U}}
\newcommand{\K}{\mathbb{K}}
\newcommand{\N}{\mathbb{N}}
\newcommand{\M}{\mathrm{M}}
\newcommand{\DL}{\mathrm{DL}}
\newcommand{\rg}{\mathrm{rg}\,}
\newcommand{\id}{\mathrm{id}}
\newcommand{\GL}{\mathrm{GL}}
\newcommand{\card}{\mathrm{Card}\,}
\newcommand{\Det}{\mathrm{Det}}
\newcommand{\union}{\cup}
\renewcommand{\Im}{\mathrm{Im}\,}
\renewcommand{\Re}{\mathrm{Re}\,}
\newcommand{\Ker}{\mathrm{Ker}\,}
\newcommand{\vect}{\mathrm{vect}}
\newcommand{\inter}{\cap}
\newcommand{\ch}{\mathrm{ch}\,}
\newcommand{\sh}{\mathrm{sh}\,}
\renewcommand{\th}{\mathrm{th}\,}
\newcommand{\argch}{\mathrm{argch}\,}
\newcommand{\argsh}{\mathrm{argsh}\,}
\newcommand{\argth}{\mathrm{argth}\,}
\newcommand{\Z}{\mathbb{Z}}
\newcommand{\mfrac}[2]{\genfrac{}{}{0pt}{}{#1}{#2}}
\newcommand{\cotan}{\mathrm{cotan}\,}
\newcommand{\tr}{\mathrm{Tr}\,}
\)
Savoir faire : Établir la relation de récurrence \(u_{n+1}=f(u_n)\), utiliser les points fixes de \(f\), prouver la convergence de \(u\).
Exercice : Soit \(\alpha\in\R_+^*\). On pose \(u_1=\sqrt{\alpha}\) puis \(u_2=\sqrt{\alpha+\sqrt{\alpha}}\) et pour tout \( n\in\N^*\),
\[u_n=\underbrace{\sqrt{ \alpha + \sqrt{ \ \cdots\ + \sqrt{\alpha} } }}_{n-1 \text{ fois l’opérateur }+}\]
- Pour tout \(n\in\N^*\), exprimer \(u_{n+1}\) en fonction de \(u_n\). En déduire que si \( (u_n)_{n\in\N^*} \) converge, ce ne peut être que vers \(l=\frac{1}{2}\big( 1+\sqrt{1+4\alpha} \big) \).
- Montrer qu’il existe \(K>0\) tel que : \( \forall n\in\N^*,\ |u_{n+1}-l|\leqslant K\, |u_n-l| \). Conclure.